Page 77 - Revised Maths Wisdom Class - 6

Fractions 75

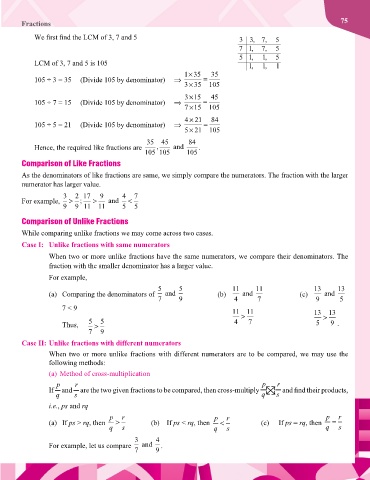
We first find the LCM of 3, 7 and 5 33, 7, 5
71, 7, 5
51, 1, 5
LCM of 3, 7 and 5 is 105 1, 1, 1
135× 35
105 ÷ 3 = 35 (Divide 105 by denominator) ⇒ =
335× 105

315× 45
105 ÷ 7 = 15 (Divide 105 by denominator) ⇒ =
715× 105

421× 8 4
105 ÷ 5 = 21 (Divide 105 by denominator) ⇒ =
521× 105
35 45 84
Hence, the required like fractions are , and .
105 105 105

Comparison of Like Fractions
As the denominators of like fractions are same, we simply compare the numerators. The fraction with the larger
numerator has larger value.
3 2 17 9 4 7
For example, > ; > and <
9 9 11 11 5 5
Comparison of Unlike Fractions

While comparing unlike fractions we may come across two cases.
Case I: Unlike fractions with same numerators

When two or more unlike fractions have the same numerators, we compare their denominators. The
fraction with the smaller denominator has a larger value.
For example,
5 5 11 11 13 13
(a) Comparing the denominators of and (b) and (c) and
7 9 4 7 9 5
7 < 9 11 11 13 13
>
Thus, 5 > 5 4 7 5 > 9 .
7 9

Case II: Unlike fractions with different numerators
When two or more unlike fractions with different numerators are to be compared, we may use the
following methods:
(a) Method of cross-multiplication
p r p r
If and are the two given fractions to be compared, then cross-multiply and find their products,
q s q s

i.e., ps and rq
p r p r p r
(a) If ps > rq, then > (b) If ps < rq, then < (c) If ps = rq, then =
q s q s q s

3 4
For example, let us compare and .
7 9