Page 109 - Revised Maths Wisdom Class - 6

Perimeter and Area 107

= s + s + s = 3s A
Hence, perimeter of an equilateral triangle = 3 × length of each side
Similarly, perimeter of any regular polygon will be: s s

n × length of each side B s C
Where ‘n’ is number of sides of the polygon.

Units of Perimeter
As the unit of length is cm, m or km and perimeter is the sum of the lengths of a closed figure, the unit of perimeter
will also be cm, m or km. Also, when we fence the fields, gardens or playground, build a racing track or walls
around the buildings, we use the concept of perimeter.

Rememb
Remember!er!
› To determine the perimeter, it is essential that the measuring unit of all sides must be same.

ILLUSTRATIONS

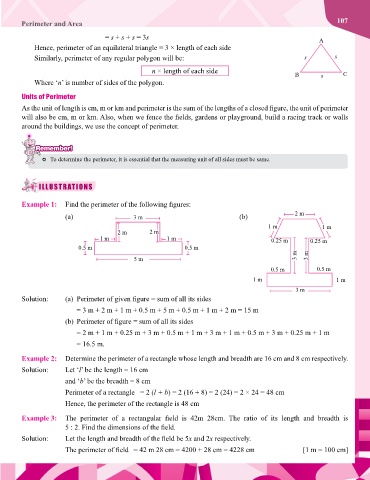
Example 1: Find the perimeter of the following figures:

(a) 3 m (b) 2 m
1 m 1 m
2 m 2 m
1 m 1 m 0.25 m 0.25 m
0.5 m 0.5 m

5 m 3 m 3 m
0.5 m 0.5 m
1 m 1 m
3 m
Solution: (a) Perimeter of given figure = sum of all its sides
= 3 m + 2 m + 1 m + 0.5 m + 5 m + 0.5 m + 1 m + 2 m = 15 m
(b) Perimeter of figure = sum of all its sides

= 2 m + 1 m + 0.25 m + 3 m + 0.5 m + 1 m + 3 m + 1 m + 0.5 m + 3 m + 0.25 m + 1 m
= 16.5 m.

Example 2: Determine the perimeter of a rectangle whose length and breadth are 16 cm and 8 cm respectively.
Solution: Let ‘l’ be the length = 16 cm

and ‘b’ be the breadth = 8 cm
Perimeter of a rectangle = 2 (l + b) = 2 (16 + 8) = 2 (24) = 2 × 24 = 48 cm
Hence, the perimeter of the rectangle is 48 cm

Example 3: The perimeter of a rectangular field is 42m 28cm. The ratio of its length and breadth is
5 : 2. Find the dimensions of the field.
Solution: Let the length and breadth of the field be 5x and 2x respectively.

The perimeter of field = 42 m 28 cm = 4200 + 28 cm = 4228 cm [1 m = 100 cm]